多水平统计模型 第10章Word格式文档下载.docx

多水平统计模型 第10章Word格式文档下载.docx

《多水平统计模型 第10章Word格式文档下载.docx》由会员分享，可在线阅读，更多相关《多水平统计模型 第10章Word格式文档下载.docx（15页珍藏版）》请在冰豆网上搜索。

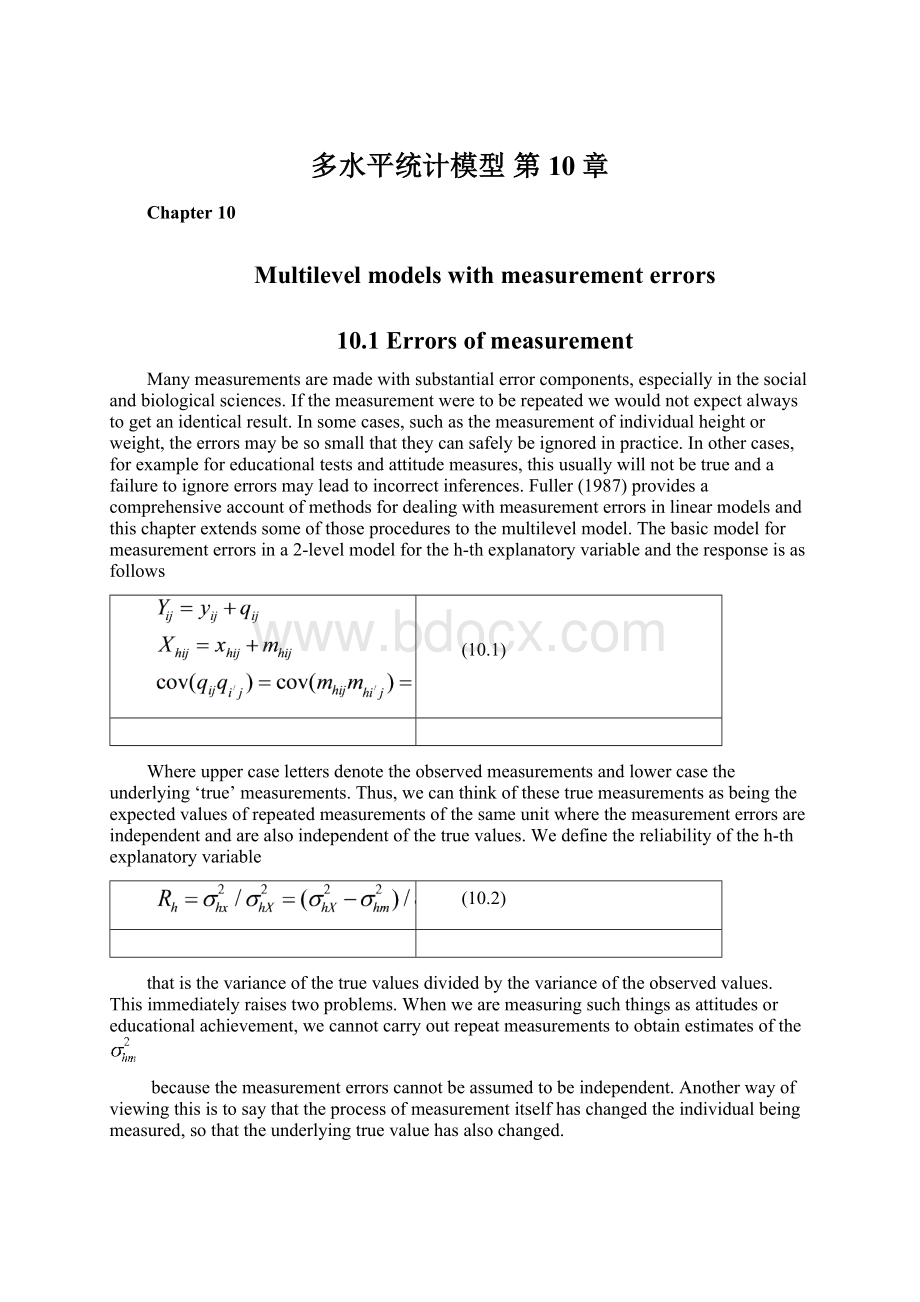
becausethemeasurementerrorscannotbeassumedtobeindependent.Anotherwayofviewingthisistosaythattheprocessofmeasurementitselfhaschangedtheindividualbeingmeasured,sothattheunderlyingtruevaluehasalsochanged.

Thesecondproblemisthatwehavetodefineasuitablepopulation.Thedefinitionofreliabilityispopulationdependent,sothatforexample,ifthemeasurementerrorvariance

remainsconstantbutthepopulationheterogeneityofthetruevaluesincreasesthenthereliabilitywillincrease.Thus,thereliabilitymaybelowerwithinpopulationsubgroups,definedbysocialstatussay,thaninthepopulationasawhole.Inparticular,thereliabilityofatestscoremaybesmallerwithinlevel2units,sayschools,thanacrossallstudents.

Inthischapterweshallassumethatthevariancesandcovariancesofthemeasurementerrorsareknown,orratherthatsuitableestimatesexists.Thetopicofmeasurementerrorestimationisacomplexone,andthereareingeneralnosimplesolutions,exceptwheretheassumptionofindependenceoferrorsonrepeatedmeasuringcanbemade.Thecommonprocedure,especiallyineducation,ofusing‘internal’measuresbaseduponcorrelationalpatternsoftestorscaleitems,isunsatisfactoryforanumberofreasonsandmayoftenresultinreliabilityestimateswhicharetoohigh.EcobandGoldstein（1983）discusstheseandproposesomealternativeestimationprocedures.McDonald（1985）andotherauthorsdiscusstheexplorationandestimationofmeasurementerrorvarianceswithinastructuralequationmodel,whichhasmuchincommonwiththesuggestionsofEcobandGoldstein（1983）.Becauseestimatesofmeasurementerrorvariancearegenerallyimpreciseitisusefultostudytheeffectsofvaryingthemandwewillillustratethisinexamples

10.2Measurementerrorsinlevel1variables

Weuseatwolevelmodeltoshowhowmeasurementerrorscanbeincorporatedintoananalysis.AfullderivationisgiveninAppendix10.1.Wewriteforthetruemodel

（10.3）

wherefornowweassumethattheexplanatoryvariablesfortherandomvariablesaremeasuredwithouterrorwhichwillbetrueforvariancecomponentmodels.Weassumethatitisthistruemodelforwhichwewishtomakeestimates.Insomesituations,forexamplewherewewishsimplytomakeapredictionforaresponsevariablebaseduponobservedvaluesthenitisappropriatetotreatthesewithoutcorrectingformeasurementerrors.Ifwewishtounderstandthenatureofanyunderlyingrelationships,however,werequireestimatesfortheparametersofthetruemodel.

Fortheobservedvariables（10.3）gives

（10.4）

InAppendix10.1weshowthatthefixedeffectsareestimatedby

（10.5）

where

isthecovariancebetweenthemeasurementerrorsforexplanatoryvariables

forthei-thlevel1unit.Thelastexpressionin（10.5）isacorrectionmatrixforthemeasurementerrorsandhaselementswhichareweightedaveragesofthecovariancesofthemeasurementerrorsforeachleveloverallthelevel1unitsinthesamplewiththeweightsbeingthediagonalelementsof

.Invariancecomponentmodelsthisisasimpleaverageoverthelevel1units,andinthecommoncasewherethecovariancematrixofthemeasurementerrorsisassumedtobeconstantoverlevel1unitswehave

（10.6）

AnapproximationtothecovariancematrixoftheestimatesisgiveninAppendix10.1asisanexpressionfortheestimationoftherandomparameters.Fortheconstantmeasurementerrorcovariancecasewithnomeasurementerrorsintheresponsevariablethiscovariancematrixisgivenby

（10.7）

andintheestimationoftherandomparameterstheterm

issubtractedfrom

ateachiteration.Itisimportantinsomeapplicationstoallowthemeasurementerrorvariancestovaryasafunctionofexplanatoryvariables.Forexample,inperinatalstudies,themeasurementofgestationlengthmaybequiteaccurateforsomepregnancieswherecarefulrecordsarekeptbutlesssoinothers.

WheretheexplanatoryvariableshaverandomcoefficientstheaboveresultsaremodifiedsomewhatandthedetailsaregiveninAppendix10.1.

10.3Measurementerrorsinhigherlevelvariables

Wherevariablesaredefinedatlevel2orabovewithmeasurementerrorswehaveanalogousresults,withdetailsgiveninAppendix10.1.Thusthecorrectiontermtobeusedinadditionto

withaconstantmeasurementerrorcovariancematrixina2-levelmodelis

（10.8）

isavectorofonesoflengthnand

isthej-thblockofV.

Acaseofparticularinterestiswherethelevel2variableisanaggregationofalevel1variable.Woodhouseetal（1995）considerthiscaseindetailandgivedetailedderivations.Considerthecasewherewehavealevel2variablewhichisthemeanofalevel1variable

Thevarianceoverthewholesampleisthereforegivenby

（10.9）

whereweassumeconstantvariancesandcovarianceswithinlevel2unitsforthe

.Thenumberoflevel1unitsactuallymeasuredinthej-thlevel2unitis

outofatotalnumberofunits

.Straightforwardestimatesoftheparameterscanbeobtainedbycarryingoutavariancecomponentsanalysiswith

asresponse,fittingonlytheoverallmeaninthefixedpart,sothatthecovarianceisthelevel2varianceestimate.

Forthetruevalueswehaveananalogousresultwherenowweconsiderthevarianceofthemeanofthetruevaluesforallthelevel1unitsineachlevel2unit.Thereare,ineffect,twosourcesoferrorin

.Thereistheerrorinherentinthelevel1measurement

whichisaveragedacrossthelevel1unitsineachlevel2unitandthereisthesamplingerrorwhichoccurswhen

thatisnotalltheunitsinthelevel2unitaremeasured.Thusthetruevalueistheaverageforallthelevel1unitsineachlevel2unitofthetruelevel1measurements.Sincethemeasurementerrorsareassumedindependentwehave

（10.10）

Thisgivesusthefollowingexpressionfortherequiredmeasurementerrorvariancefortheaggregatedvariable

（10.11）

wherethereliability

isestimatedfromthelevel1variation.

Ifboththelevel1observedvariableanditsaggregateareincludedasexplanatoryvariablesthenclearlytheirmeasurementerrorsarecorrelatedandthecorrelationisgivenby

.

Intheexpressionsforthecorrectionmatrices,wehaveconsideredtheseparatecontributionsfromlevels1and2.Wherethereisa‘cross-level’correlationbetweenmeasurementerrorsasabovethenweaddthelevel1variableto

using（10.11）forthecovariancetogetherwithazerovariance.Themeasurementerrorvarianceforthelevel1explanatoryvariablebecomesacomponentof

.AdetailedderivationoftheseresultsisgivenbyWoodhouseetal（1995）.

Table10.1ElevenyearNormalisedmathematicsscorerelatedto8yearscore,genderandsocialclassfordifferenteightyearscorelevel1reliabilities;

adjustingformeasurementerrorsatlevel1only.

Parameter

A（R1=1.0）

B（R1=0.9）

C（R1=0.8）

Fixed

Estimate（s.e.）

Intercept

0.14

0.11

0.08

8yearscore

0.095（0.0037）

0.107（0.0042）

0.122（0.0050）

Gender

-0.044（0.050）

-0.043（0.052）

NonManual

0.15（0.06）

0.11（0.06）

0.06（0.06）

Random

0.081（0.023）

0.081（0.024）

0.082（0.024）

0.423（0.023）

0.374（0.023）

0.311（0.025）

Intra-schoolcorrn.

0.16

0.18

0.21

10.4A2-levelexamplewithmeasurementerroratbothlevels.

WeusetheJuniorSchoolProjectdatareadingscoreattheageofelevenyearsasourresponsewiththeeightyearmathematicsscoreaspredictor,fittingalsosocialclass（NonmanualandManual）andgender.ThescoresatageelevenhavebeentransformedtohaveastandardNormaldistribution.Inadditionweshallallowformeasurementerrorsinboththetestscores.Thereareatotalof728studentsin48schoolsinthisanalysis.

Intheoriginalanalysesofthesedata（Mortimoreetal,1988）reliabilitiesarenotgiven,andforthereasonsgivenaboveareunlikelytobewellestimated.Forthepurposeofouranalysesweinvestigatearangeofreliabilitiesfrom0.8to1.0tostudytheeffectofintroducingincreasingamountsof